• Lesson 3 Student Document (M-A1-1-3_Lesson 3 Student Document.doc) is an explanation of the significance of the quadratic formula and why it is meaningful for the individual student. Responses will vary, but should include the generalizability of the quadratic formula and its capacity to represent numbers that do not include integers or rational and irrational numbers.

• Observations of students’ note-taking are useful for evaluating how well students are understanding and representing the quadratic formula. Look for examples that reflect general principles, such as the Zero Product Multiplication Property. Ask students to explain in their own words what they have written.

“In another lesson, we worked on solving quadratic equations by factoring.”

Display for the class: x² + 11x + 15 = 0

“Suppose we were attempting to solve the given problem. Based on yesterday’s lesson, we would attempt to solve this by factoring, so let’s give it a try.”

Direct students to attempt to solve the problem as they did during Lesson 2. Students will eventually become stuck when attempting to do the problem because this is a prime polynomial. Take a moment to discuss this with the class.

“As many of you have discovered, this quadratic equation cannot be factored. When attempting to factor we get stuck and cannot finish the problem. This is what we call a prime polynomial, which is similar to a prime number, a number whose only factors are 1 and itself.”

“So what are we going to do?”

“As it turns out, there is a mathematical formula that we can use to solve any quadratic function. This formula will work regardless of whether or not the polynomial is factorable or prime. Therefore, we have a way to solve any quadratic function!”

“Now, let’s take a look at another situation where factoring will not work.” Display the following scenario for students:

A person springs off a diving board that is 9.8 meters from the surface of the water at an initial velocity of 6.7 meters per second. This can be modeled by the equation s(t) = -4.9t² + 6.7t + 9.8, where s(t) is the height, in meters, above the surface and time (t) is in seconds. According to the equation, when will the diver reach the surface of the water? At what height is the diver after 1 second?

“Decimals make it difficult to try to factor this equation; therefore, we will need to use another method. We will attempt to solve this equation later in our lesson.” The solution is shown further on in the lesson.

Display the following notes and examples for students to learn about the quadratic formula. (Visual and auditory learners can see and hear the process of using the quadratic formula.)

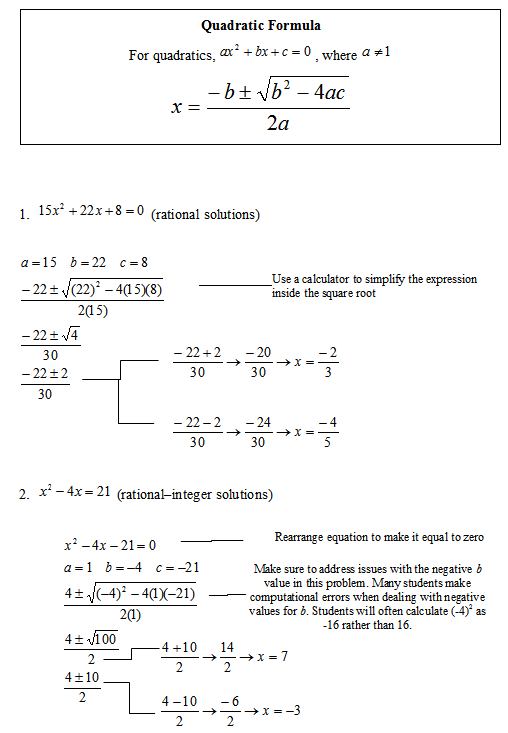
***Note: At this time you may want to point out the relationship between solving quadratics that yield integer or fraction solutions using the quadratic formula and solving quadratics by factoring. Examples 1 and 2 can be solved either way, while examples 3 and 4 that follow cannot be solved by factoring. Remind students to remember “all over 2a.”

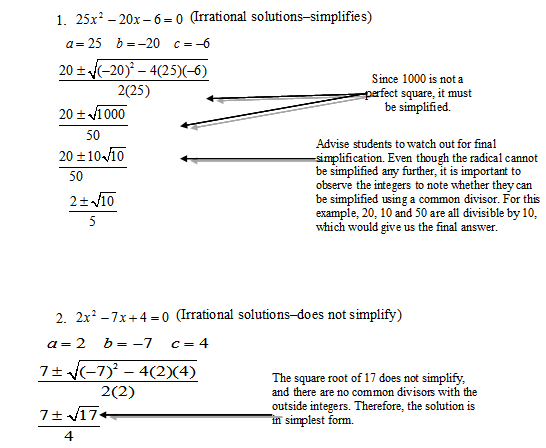
In real numbers, if the radicand is negative, students should check their calculations.

Revisit the diving problem earlier in the lesson: set the equation equal to 0 (the surface of the pool is 0 feet) and use the quadratic formula to solve. t = –0.89s or t = 2.25s. Discuss that 2.25 seconds is the reasonable answer in the situation, that –0.89s represents the time the diver starts off the platform, and that it is negative because the diver actually starts at 9.8 meters above the surface, not at the surface.

Optional: using a graphing calculator or computer software, model the graph of the equation and discuss how the visual representation corresponds to the situation. The answer to question 2 is 11.6 meters above the surface, 1.8 meters above the diving platform.

Independent Practice Activity (Intrapersonal)

After explaining the problems/examples to students, give them a chance to practice the skills on their own. The problems range from simplest to most advanced. If there is time to do this in class, do the activity as follows:

1. Print out enough copies of the Quadratic Formula Independent Practice Worksheet for each student (M-A1-1-3_Quadratic Formula Independent Practice and KEY.doc).

2. Cut the worksheet into strips so there is only one problem per strip.

3. Give each student a strip containing only problem #1.

4. Have students work through problem #1 on their own, using their notes as a reference or asking you questions as needed.

5. When students are finished with the first problem, have them check their answers with you (or put the answer key in a specific location if you do not want students to wait.)

6. If students get the answer correct, they should move to problem #2.

7. Continue this process until each student makes it through problem #4.

8. Conclude the activity by addressing any consistent errors and answering questions students had throughout the activity.

If there is not time to do this activity in class, give students the problems for homework. If any students do not finish all four problems in class, have them finish the rest for homework. Go over any problems/questions when students return the next day.

Exit Ticket: At the conclusion of the lesson, direct students to write a brief summary of why the quadratic formula is important. Students should understand that the quadratic formula is necessary to solve prime quadratics, and that it can be used as a “catch-all” method to solve any quadratic function. You may choose to have students share their thoughts with a partner or in small groups and/or have students share with the class to ensure that all are reminded of why the quadratic formula is important.

Day 2 Activity: Concept Ladder (approximately 30–45 min.)

Use the following activity as a follow-up to the lesson on the quadratic formula. The structure of this activity is designed to let you observe the work of each student as well as provide students with immediate and personalized feedback on their work. Students work at their own pace to ensure they understand each step in the process.

• Prior to the Activity

1. Open the Concept Ladder Quadratic Formula spreadsheet (M-A1-1-3_Concept Ladder Quadratic Formula.xls).

2. Print a copy of the “Record Sheet” page for each student.

3. You will also be using the Concepts page located in this same spreadsheet. Before printing or making copies of this page, insert an equation in the Concept 2 box. This is left blank to allow for multiple equations/uses. (For this first practice, use the equation 2x² - 18x + 9 = 0, or make up your own. This equation involves radicals and multiple simplifications.) Print Concepts pages for all students in the class, but do not give them to students. Cut out the concept boxes and separate them into envelopes or piles for each concept.

• During the Activity

1. Pass out the ladder page to each student. Before passing out Concept 1, explain the activity directions.

2. Explain to students, “During this activity you will be working through a problem using the quadratic formula. You will all be given a slip of paper that says ‘Concept 1’ and gives you a task to complete. You are to complete this task in the ‘concept 1’ box on the handout you just received. When you have completed Concept 1, check the accuracy of your work. If you did it correctly you will receive Concept 2 and repeat the process until you have completed all stages. If you do a step incorrectly, recheck your work.”

3. As students form a line, correct their work. Use this as an opportunity to give feedback to individual students based on their needs. Use your knowledge of your students to personalize feedback, which will provide the greatest benefit.

• After the Activity

1. When a student completes the final concept, hand him/her the Quadratic Formula Follow-up Worksheet (M-A1-1-3_Quadratic Formula Follow-up Worksheet and KEY.doc).

2. Direct students to use the slips of paper with the concepts as a way to guide them through the problems on the worksheet. Explain that they can spread the steps out on their desk and refer to them as they go through each problem.

3. If students do not finish problems in class, this is a good worksheet for them to finish for homework.

Example Problem: You have to make a square-based box without a lid, with a height of three inches and a volume of approximately 42 cubic inches. You will be taking a piece of cardboard, cutting three-inch squares from each corner, scoring between the corners, and folding up the edges. What should be the dimensions, to the nearest quarter inch, of the cardboard?

Solution: 2.26 or 9.74, but 9.74 is the only solution that actually works in the context. Rounded to the nearest quarter inch: 9.75 inches on each side.

Extension:

Use the following strategies to tailor the lesson to meet the needs of your students throughout the year.

• Routine: Use the Lesson 3 Student Document (M-A1-1-3_Lesson 3 Student Document.doc) to give students a structured format for taking notes. Provide this resource to students, as needed, to allow them to keep more organized and structured notes.

• Logical/Sequential/Visual Learners: It may be helpful for some students to see a step-by-step listing of the process used when applying the quadratic formula. Print a copy of the Concept Ladder Quadratic Formula (M-A1-1-3_Concept Ladder Quadratic Formula.xls) to give to any students who may benefit from seeing the steps written out.

• Alternate Method: (For the follow up activity–concept ladder) This activity can be modified to allow for multiple equations rather than having students work on the same equation. It is possible to shorten the ladder and list of concepts to accommodate simpler quadratic equations, such as those that do not simplify as much or that have rational solutions instead of square roots. The original activity is designed to guide students through the most difficult form of equations that involve radicals and various simplifications.

• Have students create a box out of paper using the Example Problem.

• Technology Extension: http://www.tc3.edu/instruct/sbrown/ti83/quadrat.htm: This program can be written into the program section on the graphing calculator; it will solve quadratic equations. This activity may be more appropriate for students who are at or going beyond the standards.